Introduction to Hooke's Law
What is Hooke's Law?
- Up to now, strings have been modelled as inextensible
- they cannot stretch (inelastic)
- we assume that the tension measured at any point along the string is the same constant value
- Things that stretch (or compress, e.g. springs) are called elastic
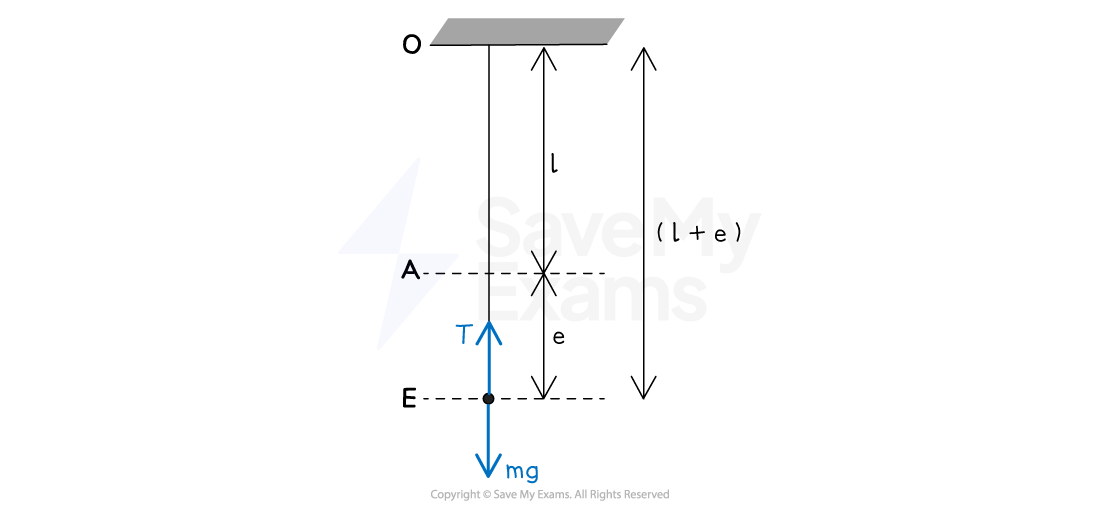
- Imagine two elastic strings held taut and at rest, but with one stretched further than the other
- measuring the tension at different points along one string gives the same value,
- but that "value" will be higher for the more stretched string than for the less stretched string
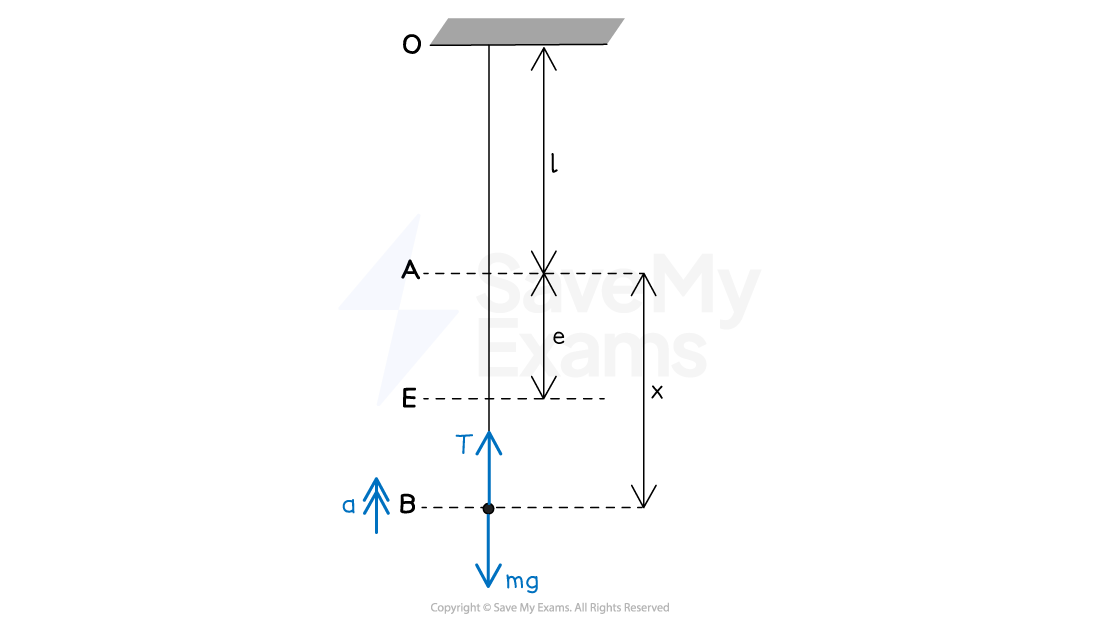
- Hooke's Law tells us that the value of tension,
N, depends on how far it's been stretched (the extension,
metres) beyond its natural (unstretched) length (
metres)
- The law is
- where
is the modulus of elasticity, with units of Newtons,
- it measures the stiffness of the material the string (or spring) is made from,
- the higher
is, the stiffer the string / spring is
- The law is
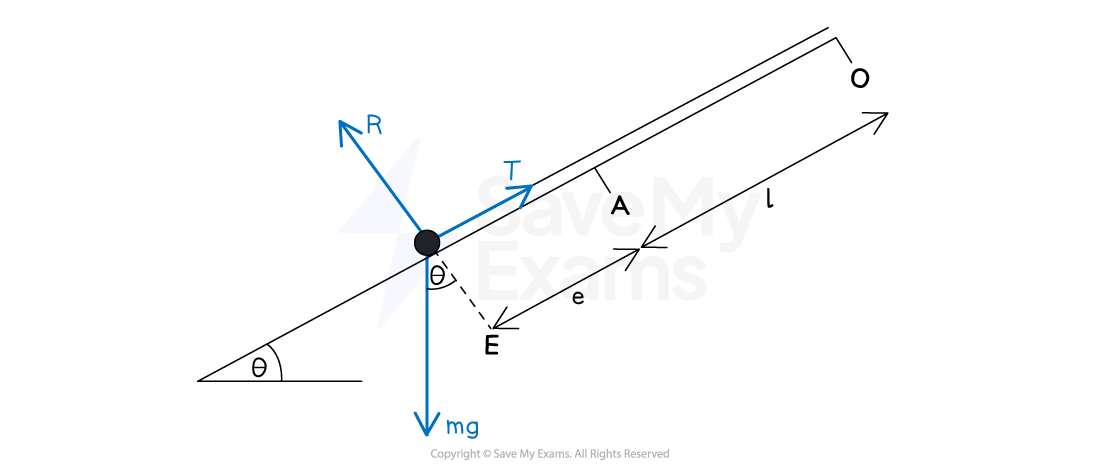
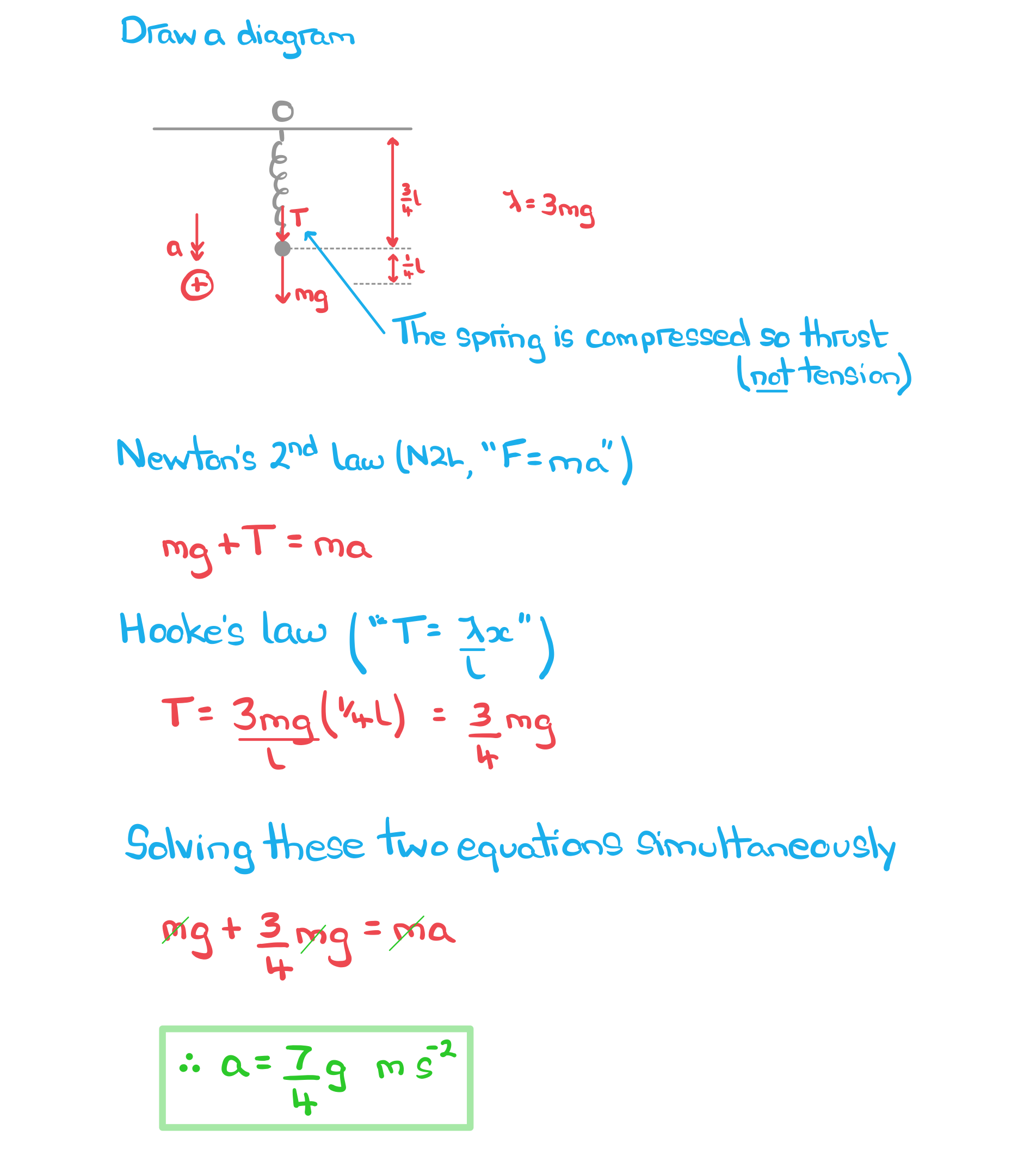
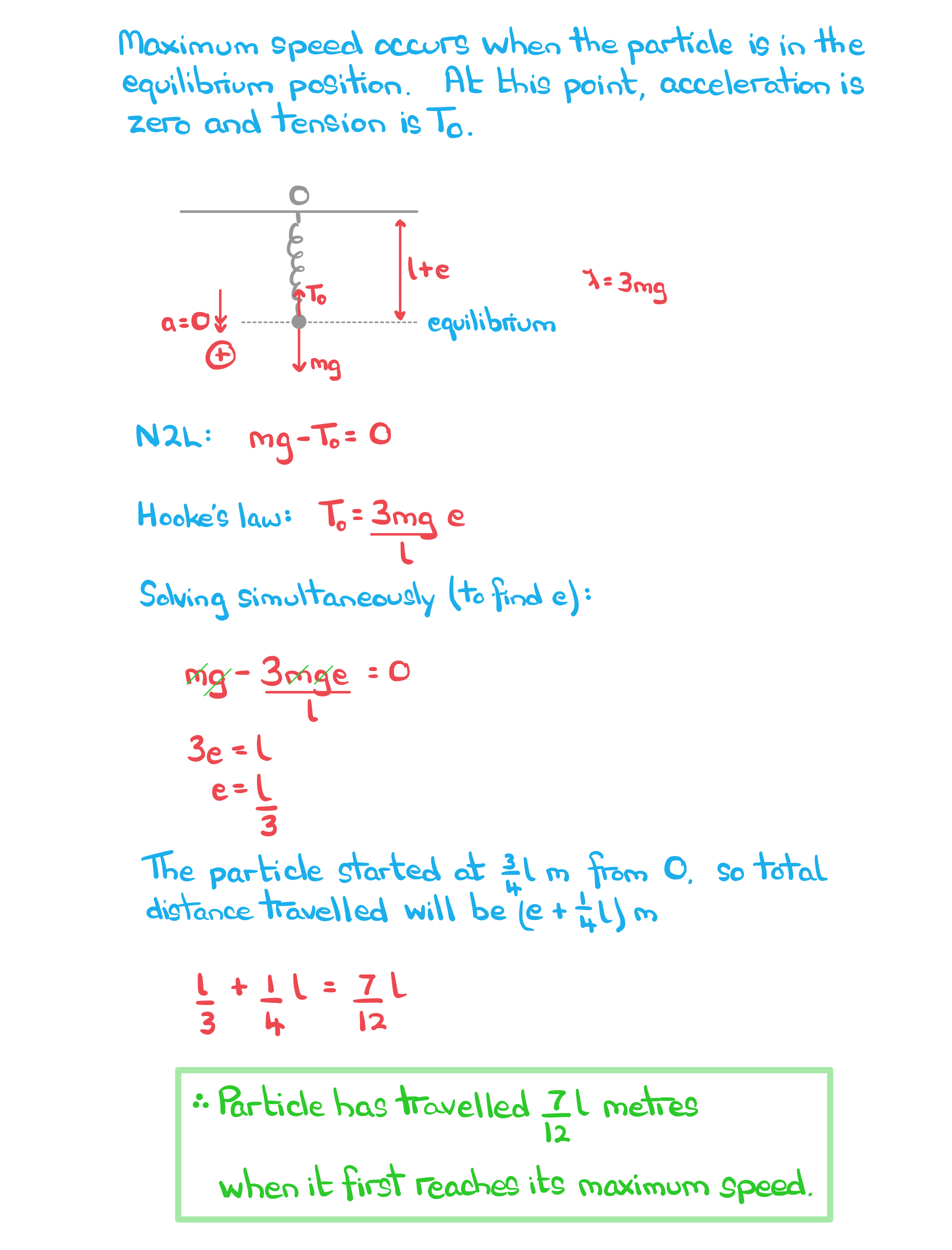
- Springs can be compressed but elastic strings can't (they'd go slack)
- Hooke's Law works for compression of springs too
- Instead of measuring extension,
measures the length of compression (from its natural length)
- just make sure any tension arrows reverse direction to be compression (thrust) arrows!
Exam Tip
- In more algebraic questions, the modulus of elasticity may be given in the form
Newtons, where
is a constant
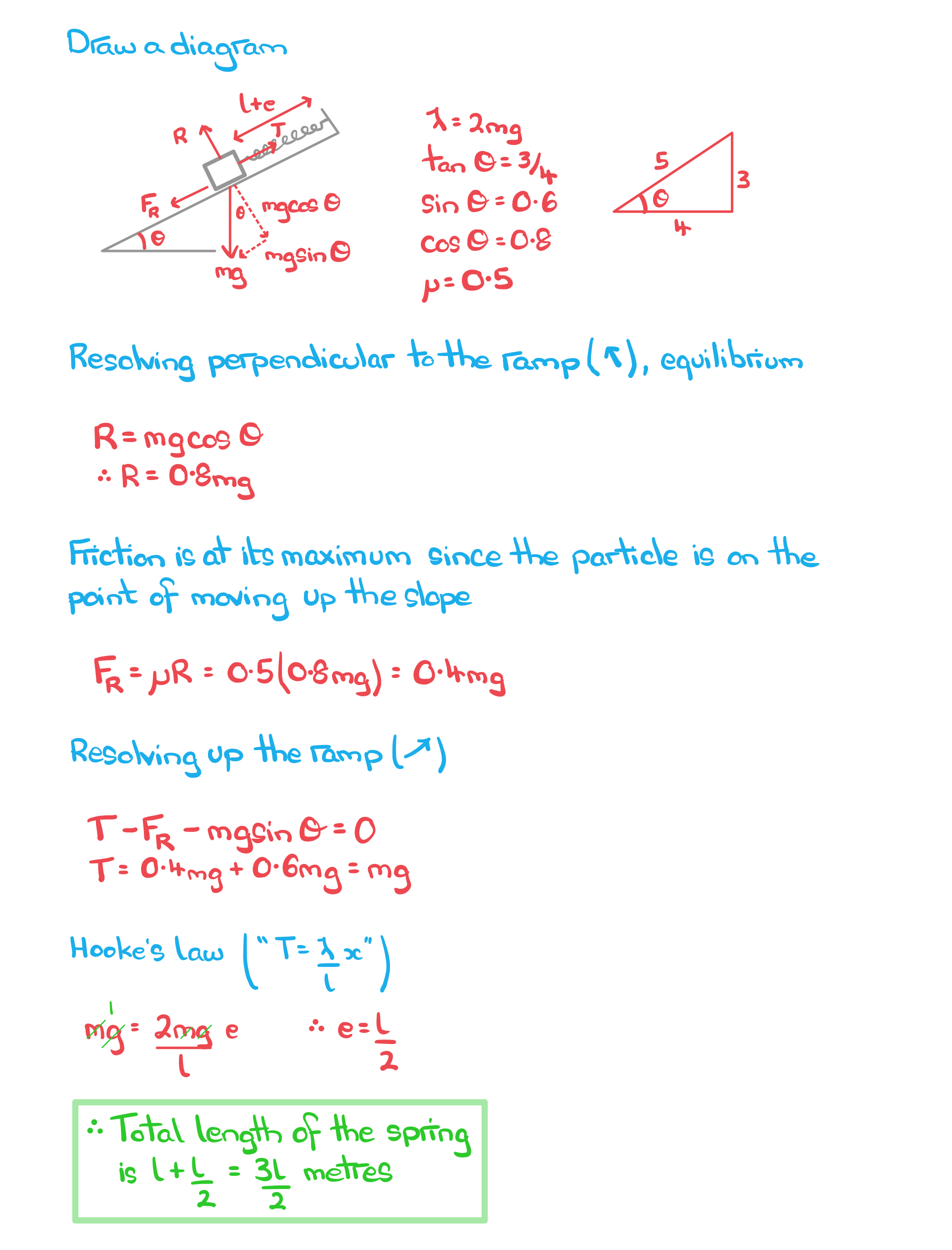
Worked example
An elastic string of natural length metres and modulus of elasticity 20 N is stretched to a total length of
metres.
Find the tension in the string.