Problem Solving with Oblique Collisions (Edexcel A Level Further Maths: Further Mechanics 1)

Revision Note

Test Yourself

Author

Jamie W

Expertise

Maths

Energy in 2D Collisions

How do I find the kinetic energy loss from a collision?

A common question, usually as a follow up to a collisions question, is to find the kinetic energy lost as a result of the impact
If $e = 1$ kinetic energy is conserved in the collision
- If $e < 1$ there will be a decrease in the total kinetic energy of the two particles
Recall that kinetic energy can be calculated using $K . E . = \frac{1}{2} m v^{2}$
As the velocity is squared, its sign does not affect the kinetic energy
- Hence, $v^{2}$ here is effectively the speed squared
When dealing with motion in two dimensions, the velocity may be described in two components, e.g. $v = (3 i - 4 j) {ms}^{- 1}$
- To use this with $K . E . = \frac{1}{2} m v^{2}$ , the magnitude of the vector must be found, using Pythagoras
  - In this case $|v| = \sqrt{3^{2} + 4^{2}} = 5 {ms}^{- 1}$
  - If $m = 7 kg$ then $K . E . = \frac{1}{2} \times 7 \times 5^{2} = 87.5 J$
To find the loss in kinetic energy due to a collision, find the difference between the kinetic energy before the collision, and the kinetic energy after the collision
- The question may ask to find the loss in kinetic energy for one particular particle,
  - or it could ask to find the total loss in kinetic energy for both particles

Exam Tip

When finding the speed from two components, your working will look like this: $v = \sqrt{x^{2} + y^{2}}$
- When finding kinetic energy, the speed is squared, so your working may look like this: $\frac{1}{2} m {(\sqrt{x^{2} + y^{2}})}^{2}$
- It can be quicker to not do the square rooting part if you know you are only using it to find $v^{2}$
- e.g. $\frac{1}{2} m (x^{2} + y^{2})$
You can also use the scalar product to find the kinetic energy:
- $\frac{1}{2} m (v \cdot v)$ where $v$ is the vector form of the velocity

Worked example

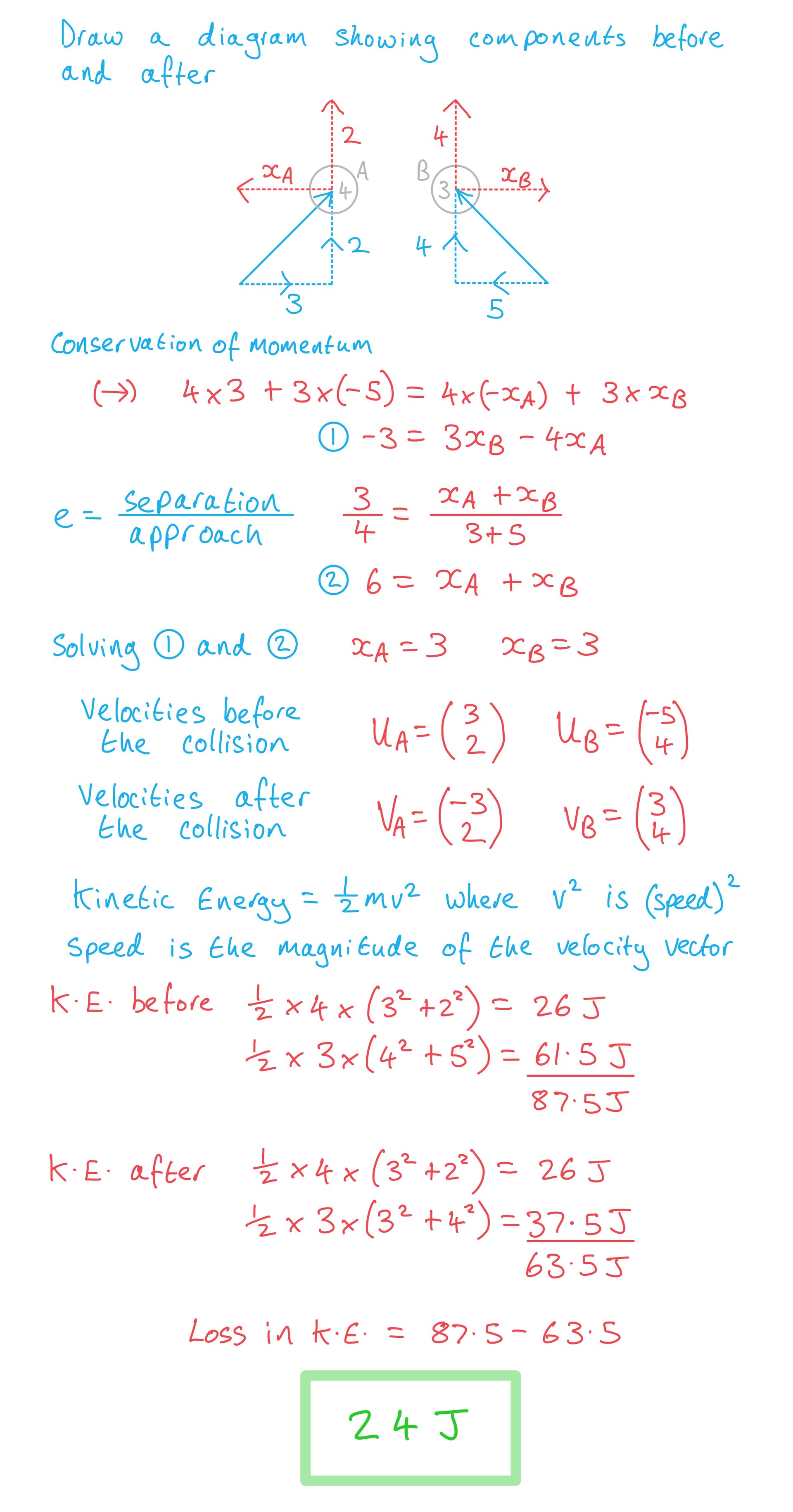
A smooth sphere A of mass 4 kg is moving on a smooth horizontal surface with velocity $(3 i + 2 j) {ms}^{- 1}$ . Another smooth sphere B of mass 3 kg and the same radius as A is moving on the same surface with velocity $(- 5 i + 4 j) {ms}^{- 1}$ . The spheres collide when their line of centres is parallel to $i$ . The coefficient of restitution between the spheres is $\frac{3}{4}$ .
Find the kinetic energy lost in the impact in total.

worked example showing how to find the loss in kinetic energy after 2 spheres collide, with velocities in 2 dimensions

Angles of Deflection

How do I find the angle of deflection after collision with a surface?

Once the speed and direction of a sphere after a collision have been calculated, a common follow up question is to find the angle of deflection
The angle of deflection is the angle by which the path of the object has changed from its original trajectory
To find the angle of deflection it is helpful to sketch a new diagram, with only the angles marked on it
- The diagram below shows a particle which collides with the surface at angle $α °$ and leaves the surface at angle $β °$
Draw a dashed line showing the continuing path of the object, if it had not collided with the surface
Use vertically opposite angles to mark the other angle which is equal to $α °$
It can now be seen that the angle of deflection, the angle by which the path of the object has turned,
- is equal to $(α + β) °$

diagram of a rebound with a wall, showing the angle of deflection

How do I find the angle of deflection after a collision between two spheres?

Exactly the same concept described for finding the angle of deflection after colliding with a surface, applies when two spheres collide
- The "surface" when two spheres collide, is the common tangent line between the two spheres
The angle of deflection is still the angle by which the path of the object has changed from its original trajectory
The main difference with spheres, is that the angles may be marked in varying ways on the diagram depending on the problem
To deal with this draw a diagram clearly showing:
- the velocity of the sphere before and after the collision,
- a dashed line showing the continuing path of the object, if it had not collided with the other sphere,
- any angles that were given or have been calculated,
- and the common tangent line of the spheres
Remembering that the angle of deflection is the angle between the continued original path of the sphere, and its new path, find any missing angles on the diagram using:
- vertically opposite angles are equal,
- angles on a straight line sum to 180°,
- and angles in a right angle sum to 90°

diagram of an oblique collision of two spheres, showing the angle of deflection

In the above diagram, a sphere collides at an angle $α °$ above the horizontal, and after the collision has a direction which forms angle $β °$ below the horizontal
- By drawing the common tangent line, the angle $90 - α$ can be filled in, adjacent to $α$
- Similarly, $90 - β$ can be filled in, adjacent to $β$
- By using vertically opposite angles, another $90 - α$ angle can be marked on the diagram
- This then shows (in green) that the angle of deflection, the angle by which the path of the object has turned, is equal to $(90 - α) + (90 - β)$
- This simplifies to $180 - α - β$
Note that this answer does not always apply, as it depends which angles are given and marked on the diagram

How do I use the scalar (dot) product to find the angle of deflection?

The scalar product (or dot product) can also be used to find the angle of deflection
We can use the property: $a \cdot b = |a| |b| \cos θ$
If $a$ is the vector describing the velocity of the sphere before the collision,
- and $b$ is the vector describing the velocity of the sphere after the collision,
- then $θ$ will be the angle of deflection
This can then be rearranged to $θ = \cos^{- 1} (\frac{a \cdot b}{|a| |b|})$

Exam Tip

Drawing a separate diagram focusing on only the angles can be helpful, without the algebra and masses etc that you may have used earlier in the question
- You should include the velocities both before and after on the same diagram to do this

Worked example

Two small smooth spheres A and B collide when the line joining their centres is parallel to $i$ . Before the collision the velocity of A is $(3 i + 2 j) {ms}^{- 1}$ and the velocity of B is $(- 4 i + 5 j) {ms}^{- 1}$ . After the collision the velocity of A is $(- 5.4 i + 2 j) {ms}^{- 1}$ and the velocity of B is $(1.6 i + 5 j) {ms}^{- 1}$ .

Find the angle of deflection of sphere A.

worked example showing how to find the angle of deflection using geometry or the scalar product

Problem Solving with Oblique Collisions

Tips for problem solving with oblique collisions

Drawing a large, clear diagram will always help and prevent working from becoming squashed together
- Establish a routine of how you lay out your diagrams and working, so you can do it routinely and quickly in an exam
Adding an arrow, or pair of arrows, to show the direction of the impulse will help remind you which components will be affected
Fill in the things you can work out straight away
- For example the components of the velocity perpendicular to the impulse are unaffected, so you can usually fill this in immediately
Use sensible labels for velocities and components of velocities
- e.g. $x_{A}$ for the unknown horizontal component of the velocity of sphere A, $y_{A}$ for an unknown vertical component, and $v_{A}$ for the unknown final speed $(\sqrt{{x_{A}}^{2} + {y_{A}}^{2}})$ of sphere A.
If you are not sure how to find a piece of missing information, and have filled in everything you can work out by inspection, follow the usual processes to form equations which may help you
- Apply conservation of linear momentum in the direction parallel to the impulse
  - $m_{1} u_{1} + m_{2} u_{2} = m_{1} v_{1} + m_{2} v_{2}$
- Apply Newton's law of restitution in the direction of the impulse
  - $e = \frac{Speed of separation of the objects}{Speed of approach of the objects}$
- This will often lead to a pair of simultaneous equations which can be solved
- Be careful with positive and negative signs when forming these equations, the signs of the velocities will depend on how they are modelled in the diagram
  - e.g. If the arrows are drawn pointing away from each other in the "after" diagram, the speed of separation will be $x_{A} + x_{B}$
  - If the arrows were drawn both pointing to the right, the speed of separation would be $x_{B} - x_{A}$
The equation $I = m (v - u)$ , where $I$ is the impulse, can also sometimes be useful with 2D collisions
- It can be used with vectors for $I$ , $v$ , and $u$ :
  - $I = m (v - u)$
- This is particularly helpful when a question does not describe the direction of a wall, as it can be used to find the direction of the impulse, which is always perpendicular to the wall
Make use of the scalar (dot) product formulae for collisions with a surface
- $v \cdot P = - e u \cdot P$ where $P$ is the direction perpendicular to the surface
- $v \cdot W = u \cdot W$ where $W$ is the direction of the surface (or wall)
  - Remember that the direction of the wall and the direction of the impulse will be perpendicular, e.g. $(3 i + 1 j)$ and $(- 1 i + 3 j)$
- These formulae are most useful when a surface is not in a "nice" direction
  - e.g. when it is not parallel to the x or y axis when the sphere is moving in the xy-plane
You may need to consider kinetic energy before and after the collision for one or both of the objects using,
- $K . E . = \frac{1}{2} m v^{2}$

Exam Tip

Sometimes questions will be entirely algebraic,
- in this case apply the same procedures and methods as above,
- and form equations using conservation of linear momentum and Newton's law of restitution
- Label any angles on the diagram clearly and use simple angle geometry to help you
  - e.g. Vertically opposite angles are equal, angles in a right angle sum to 90°, and angles on a straight line sum to 180°
Sometimes you may need to use trigonometric identities from your pure maths knowledge to help simplify or rearrange to reach a required expression

Worked example

A smooth uniform sphere A collides with an identical sphere B which is at rest. When the spheres collide A is moving such that it forms an angle of 30° to the line joining the centres of the spheres, as shown in the diagram. The coefficient of restitution between the two spheres is $e$ . Sphere A is deflected by angle $α$ as a result of the collision, where $0 ° < α < 60 °$ .

Show that $\tan α = \frac{\sqrt{3} (1 + e)}{5 - 3 e}$ .

worked example question image showing incident angle of a sphere in an oblique collision of two spheres

worked example for an algebraic problem solving question for oblique collisions

You've read 0 of your 0 free revision notes

Get unlimited access

to absolutely everything:

Downloadable PDFs
Unlimited Revision Notes
Topic Questions
Past Papers
Model Answers
Videos (Maths and Science)

Join the 100,000+ Students that ❤️ Save My Exams

the (exam) results speak for themselves:

Test yourself

Did this page help you?

Problem Solving with Oblique Collisions (Edexcel A Level Further Maths: Further Mechanics 1)

Revision Note

How do I find the kinetic energy loss from a collision?

How do I find the angle of deflection after collision with a surface?

How do I find the angle of deflection after a collision between two spheres?

How do I use the scalar (dot) product to find the angle of deflection?

Tips for problem solving with oblique collisions

You've read 0 of your 0 free revision notes

Get unlimited access

Join the 100,000+ Students that ❤️ Save My Exams

Author: Jamie W